Serviços Personalizados
Journal
Artigo
Compartilhar
Acta Scientiarum. Education
versão impressa ISSN 2178-5198versão On-line ISSN 2178-5201
Acta Educ. vol.45 Maringá 2023 Epub 01-Dez-2023
https://doi.org/10.4025/actascieduc.v45i1.62025
TEACHERS' FORMATION AND PUBLIC POLICY
The instrument jacente no plano in a practice in the geometry teaching laboratory
1Universidade Estadual do Ceará, Av. Dr. Silas Munguba, 1700, 60714-903, Fortaleza, Ceará, Brasil.
Research in Mathematics Education has increasingly highlighted the need for a Mathematics Teaching Laboratory to be made available in Primary and Secondary Education schools and in universities. One of the justifications is that this environment favors the training of students, as in this environment they can both learn and learn how to learn. In this article, the objective is to present a laboratory practice with undergraduate Mathematics Education students on the mobilization of the concepts of isosceles right triangle and perpendicularity in space based on the construction of the instrument jacente no plano - an instrument to find the altitude of sun - highlighting its didactic potential. This action has a qualitative nature and was supported by the contribution of a Basic Problematization Units. The study reveals, for example, based on the need to position the triangle perpendicular to the board, that the action encouraged students to think about a strategy which considered material and mathematical aspects. They were also able to visualize and apply abstract concepts in practical terms, a fact that possibly favored the attribution of even more meanings to the mobilized concepts. Therefore, it is concluded that exploring the instrument in a practice with undergraduate students can contribute to their professional development.
Keywords: Geometry laboratory; instrument jacente no plano; initial teacher training
Pesquisas em Educação Matemática têm apontado caa vez mais a necessidade de um Laboratório de Ensino de Matemática ser disponibilizado em escolas da Educação Básica e em universidades. Uma das justificativas é que esse ambiente favorece a formação dos estudantes, pois neste se pode tanto aprender, como também aprender a aprender. Neste artigo, tem-se tem como objetivo apresentar uma prática laboratorial com discentes da Licenciatura em Matemática sobre a mobilização dos conceitos de triângulo retângulo isósceles e de perpendicularismo no espaço a partir da construção do instrumento jacente no plano dando destaque ao seu potencial didático. Essa ação tem cunho qualitativo e esteve amparada no aporte de uma Unidade Básica de Problematização. O estudo revela, por exemplo, a partir da necessidade de posicionamento do triângulo de forma perpendicular a tábua, que a ação impulsionou os discentes a pensar em uma estratégia levando em consideração aspectos materiais e matemáticos. Eles também puderam visualizar e aplicar conceitos abstratos em termos práticos, fato que, possivelmente favoreceu a atribuição de ainda mais significados aos conceitos mobilizados. Portanto, conclui-se que explorar o instrumento em uma prática junto a estudantes da licenciatura pode contribuir para o desenvolvimento profissional.
Palavras-chave: laboratório de geometria; instrumento jacente no plano; formação inicial de professores
La investigación en Educación Matemática ha señalado cada vez más la necesidad de disponer de un Laboratorio de Enseñanza de las Matemáticas en las escuelas y universidades de Educación Básica. Una de las razones es que este entorno favorece la educación de los estudiantes, ya que es posible aprender y aprender a aprender. En este artículo, el objetivo es presentar una práctica de laboratorio con estudiantes de la Licenciatura en Matemáticas sobre la movilización de los conceptos de triángulo rectángulo isósceles y perpendicularidad en el espacio a partir de la construcción del instrumento jacente no plano destacando su potencial didáctico. Esta acción tiene carácter cualitativo y fue apoyada con el aporte de una Unidad Básica de Problematización. El estudio revela, por ejemplo, a partir de la necesidad de posicionar el triángulo perpendicular al tablero, que la acción impulsó a los estudiantes a pensar en una estrategia teniendo en cuenta aspectos materiales y matemáticos. También fueron capaces de visualizar y aplicar conceptos abstractos en términos prácticos, hecho que posiblemente favoreció la atribución de aún más significados a los conceptos movilizados. Por tanto, se concluye que explorar el instrumento en la práctica con estudiantes de pregrado puede contribuir al desarrollo profesional.
Palabras clave: laboratorio de geometría; instrumento jacente no plano; formación inicial del profesorado
Introduction10
In the field of Mathematics Education, the Mathematics Teaching Laboratory (MTL) is seen as a suitable environment for teacher training, whether initial or continuous (Lorenzato, 2012; Turrioni, 2004). In this regard, it is known that one of the laboratory's potentials for the teaching and learning process is working with concrete materials for teaching (Lorenzato, 2012). Here attention is given to the instrument jacente no plano - which does not have a translation into English, but can be described as an instrument to find the altitude of sun - designed by Pedro Nunes (1502-1578) in the context of navigation as an alternative for determining the height of the Sun above the horizon. As according to Lorenzato (2012), measuring instruments can also be part of the MTL.
It is understood that the instrument jacente no plano can be considered a Teaching Material (MD), since a resource of this type “[...] is any instrument useful to the teaching-learning process. Therefore, MD can be chalk, a calculator, a film, a book, a puzzle, a game, a packaging, a transparency, among others” (Lorenzato, 2012, p. 18). The approximations of the instrument jacente no plano with the teacher training, which were already conducted so far, indicate that the need for students to understand the geometric concepts that are synthesized in it, whether through its construction and/or use, favors the teaching-learning process. (Oliveira, 2019; 2021).
From the discussions surrounding resources aimed at the laboratory, it is known that there is a consensus that “[...] perhaps the best potential of the MD is revealed when the MD is constructed by the students themselves, as it is during this that unforeseen events and challenges arise, which lead students to make conjectures and discover paths and solutions” (Lorenzato, 2012, p. 28). In these terms, the question arises: what is the didactic potential of the construction of the instrument jacente no plano for a practice in the Mathematics Laboratory, when focusing on the teaching and learning process of the concepts of isosceles right triangle and perpendicularity in space?
Attached to this question, in this article, the objective is to present a laboratory practice with undergraduate students of Mathematics Education on the mobilization of the concepts of isosceles right triangle and perpendicularity in space, based on the construction of the instrument jacente no plano, highlighting its didactic potential. The formative action took place in the Mathematics and Teaching Laboratory Professor Bernardo Rodrigues Torres (LAbMatEN) at the Ceará State University (UECE)11, particularly a space provided in the Geometry Teaching Laboratory discipline12.
This work, methodologically, has a qualitative basis (Creswell, 2010) and is anchored in the proposal for a Basic Problematization Unit (BPU) in the format created by Miguel and Mendes (2010). That said, immediately afterwards we show the perpendicularity in space present in the construction of the instrument, then the theoretical methodological elements that guided the research, and before the final considerations there are the results and discussions.
The instrument jacente no plano and the perpendicularity in space
It is known that it is important “[...] for the teacher to know how to use the MD correctly. Because these, like other instruments, such as the brush, the revolver, the hoe, the ball, the automobile, the scalpel, the blackboard, the lipstick, the bell, require specific knowledge from those who use them” (Lorenzato, 2012, p. 24). With regard to this conception, the instrument jacente no plano is addressed, paying attention to the perpendicularity in space that it has synthesized.
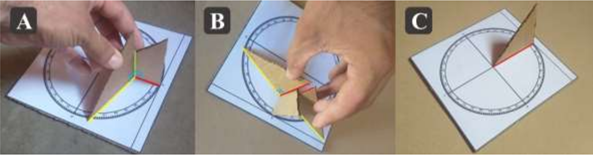
The instrument jacente no plano was released in the 16th century initially in the Petri Nonii Salaciensis Opera13 in 1566 and later in the work De arte atque ratione navigandi published in 1573, both written by Pedro Nunes14. Given the content of these means of communication, it is understood that the device was born in the context of Portuguese navigation, in which its function was to serve as another alternative for determining the height of the Sun above the horizon, as well as, for example, the nautical ring, the astrolabe, the cross-staff, and the quadrant (Oliveira & Pereira, 2020). The instrument has the following physical form (Figure 1).
The instrument is composed of a board and an isosceles right triangle placed perpendicular to the board. In this configuration shown in Figure 1, on the board there is a circumference graduated in 360º and a tangent line to it15. Regarding the use of this instrument, there is no record during its development period that indicates that it was actually used in a practical way in navigation. It is only known that it was used in theoretical classes by João Baptista de Lavanha (c.1550-1624) as we can verify on the Tratado del Arte de Navegar (Canas, 2011) and in another art of sailing from the 17th century by an unknown author (Almeida, 2011).
The absence of the instrument jacente no plano in navigation practice has been justified by the need for it to be positioned parallel to the plane of the horizon, which is difficult to achieve on the high seas. However, this justification possibly does not include the real reason for this, as it is known that there were other instruments, such as the needle, which should be parallel to the horizon and they were used on the high seas. Perhaps the non-use of the instrument jacente no plano is associated with the fact that other devices already existed, much more appreciated by navigators for determining the height of the Sun (Oliveira & Pereira, 2020).
That said, now highlighting the concept of perpendicularity in space synthesized in the apparatus, see Figure 2.
In this illustration, a way of positioning the isosceles right triangle is represented so that it is perpendicular to the board from another triangle that does not necessarily need to be right-angled and isosceles, it just needs to be right-angled. In Figure 2 (A), we can see the reason in which it is possible to state that the triangle will be perpendicular to the board that has a graduated circumference. Note, the line segment in light green is perpendicular to the plane on the board, as it is perpendicular to two concurrent line segments contained in that plane (the one in yellow and the other in red). The same argument is valid to justify the strategy in Figure 2 (B). Although, in Figure 2 (C), the triangle is positioned perpendicular to the board.
Theoretical methodological elements
This study has a qualitative basis (Creswell, 2010) and is anchored in the proposal for a Basic Problematization Unit (BPU) designed by Miguel and Mendes (2010). In this context, BPU gains space due to its bias that values work with investigative activities in the mathematics classroom based on elements/resources/sources from the history of mathematics. In these terms, the BPU meets what is expected to be provided at the MTL, that is, an environment in which exploration and investigation are key elements in the educational process (Lorenzato, 2012).
It is known that at the basis of the investigative activities involving the BPU is the historical investigation proposed by Iran Abreu Mendes (2001, 2008, 2009, 2015), which is understood to be constituted through investigative teaching activities, guided in historical information combined with a didactic approach that provides understanding of the process of constructing mathematical knowledge. In defense of historical investigation, Mendes (2001, p. 95) explains:
In order to conduct a meaningful [process of] teaching-learning in mathematics, it is necessary to use historical activities, search in the existing historical material for all useful information for conducting our teaching activities and only from there guide students to conduct the activities. However, a question arises at this point: how to conduct this process? This question is resolved when we reflect on the need to seek the historical investigation as a means of (re)constructing the mathematics produced in different socio-cultural contexts and at various times in human life.
In this sense, it is understood that it is pertinent to seek to conduct the didactic direction of the class so that the mathematical reasons that permeate the activity can be explained, that is, one must be concerned with a mathematical epistemology, with a conceptual and procedural understanding. In this way, it is understood that one can “[...] contribute to the achievement of meaningful teaching and learning of Mathematics, by involving problematizing historical situations that lead students in search of their mathematical learning” (Mendes, 2015, p. 21).
Giving particular focus to BPU, it is important to highlight that:
A BPU is nothing more than a discursive memory flash which describes a situated practice in a determined field of human activity, and it would actually have been used to answer the necessary piece of a community of practice at some point in the development of that activity in history (Miguel & Mendes, 2010, p. 386).
In these terms, it is understood that the BPU serves as a basis for the problematization which must be thought about, among other elements, according to a sociocultural practice (Farias & Mendes, 2014). In other words, the BPU can also be defined as “[...] an activity materialized by a text that clearly describes a sociocultural practice, preserving its historical and technical aspects, in addition to clarifying the authorship of the performance and techniques mobilized in it” (Martins, 2021, p. 203). As a way of developing a BPU, in addition to the fundamental references (Mendes, 2009; Miguel & Mendes, 2010), we also follow the 7 steps proposed by Martins (2021), namely: 1) present activities to students; 2) group formation; 3) distribution and managing of activity; 4) presentation and discussion of results; 5) evaluation by students; 6) evaluation (of students) by the teacher, and; 7) presentation of mathematical content.
Given this support and guidance, the activity took place in a remote synchronous form via the Zoom platform, lasting four class hours. The Thematic Unit worked on was Geometry, and its objects of knowledge were the concepts of isosceles right triangle and perpendicularity in space. The audience was fifteen students from the undergraduate Mathematics Education degree at the Ceará State University (UECE), particularly students from the Geometry Teaching Laboratory discipline16. For the development of laboratory practice, a sheet of A4 paper was given to the students, with practice guidelines.
Preliminarily, it is worth highlighting that the BPU (discursive memory flash) proposed to the students was: take on the role of a maker of nautical instruments in the 16th century. While in his workshop for another day of work, a desperate navigator arrives in the early afternoon who has a trip scheduled for India the next morning. His distress was due to the fact that some instruments that were on his ship were looted, including the astrolabe, the quadrant, and the cross-staff (instruments with which the height of the Sun above the horizon could be determined). The navigator then unwraps from a package in his hands a sheet of paper with some excerpts from a work by Pedro Nunes that the navigator came across in a class taught by João Baptista Lavanha at the Mathematics Academy in Madrid. Along with the text, the navigator also removes from the package a board that has a circumference graduated into 360 parts and a line segment tangent to the circumference, as proposed by Pedro Nunes.
That said, the navigator begs you, the instrument maker, to complete the construction of the apparatus so that he can use it the next day on his trip. To build it, follow the excerpt from Pedro Nunes (2008, p. 358):
[...] fabricate, in a hard material, a right-angled and isosceles triangle fgh, so that the sides fg and gh make a right angle and are equal to the semidiameter of the drawn circle. Then place this triangle perpendicularly to the circular board, in such a way that the side gh fits perfectly to ae, the semidiameter of the circle, that is, [in a way in which] g is with a, and h is with e; consequently, point f will be upwards.
To the development of the practice, according to the ideas of Cohen and Lotan (2017), the fifteen participants were instructed to organize themselves into groups of three students, which resulted in five simultaneous breakout rooms on Zoom. Room 1 (Isadora, Fábio, and Rebeca), Room 2 (Flora, Gabriel, and Graciliano), Room 3 (Antônio, Clara, and Francisco), Room 4 (Getúlio, Fernando, and Marcos), and Room 5 (Umberto, Luiza, and Socorro)17. Each student, in addition to developing the activity, also had a role in the progress of the proposed situation, so they were instructed that the first action they should take was to define someone to act as: 1) time controller will be responsible for alerting the group about the time; 2) facilitator will be the student responsible for promoting understanding of the task; 3) harmonizer will have the function of inhibiting, appeasing and mediating group work so that there are no conflicts that deviate from the proposed situation and; 4) reporter is responsible for exposing the internal work of their group to all participants in the activity. The criteria for defining the roles were that whoever's birthday was closest to the month of May will be the time controller; the second closest will be the facilitator; the third closest is the harmonizer) and the furthest will be the reporter. As there were only three students present in each group, it was advised that the roles of time controller and harmonizer would be the responsibility of a single participant. To construct the instrument jacente no plano, the following materials were previously requested from the students (Figure 3).

Source: Authors’ archive
Figure 3 Materials requested for the construction of the instrument jacente no plano.
As it is possible to see, the materials were only a sheet of A4 paper with a graduated circumference (a kind of template with the circumference ready to be placed on a hard material), scissors, ruler, compass, pencil, eraser, cardboard sheet (to use as a board and to make the triangle), and glue. These are all low-cost resources, which can possibly enhance the conducting of activities with the construction of the instrument jacente no plano, many of them, in fact, can already be easily acquired at school/university.
That said, it is also worth highlighting that the analysis of the practice is based on the assumptions of Laurence Bardin's Content Analysis (2011). In this way, we work with elements of the three stages that are foreseen by this method, namely: 1) pre-analysis; 2) exploration of the material; and 3) treatment of results, inference, and interpretation.
It is known that for the first stage, the orientation is to conduct a floating reading of the material, the choice of documents that will be analyzed, the constitution of the corpus, the formulation of hypotheses, and the preparation of the material (Bardin, 2011). In view of this, it is important highlighting that the material of analysis revolves around the audio and video recording of the aforementioned Geometry Laboratory practice18. From a floating reading of the video, it is organized for the analysis into two types of documents, one of which is a transcription of the practice audio and the other in the format of images of moments from the recording. It is understood that this corpus of data can benefit the development of the interpretation and inference stage. The transitions of the vocal record, as well as the images, both cover all moments of laboratory practice, that is, there are records of students during group work in each of the five virtual rooms created in Zoom.
Regarding the second phase - exploration of the material - it is worth highlighting that the direction was focused on coding and categorizing the data foreseen by the Content Analysis (Bardin, 2011). In coding, the notions of registration units and context units were used. The recording units were listed according to the research question, considering the main descriptors/keywords (in content analysis they are called words/terms) involved in the research, they were: isosceles right triangle and perpendicularity.
In relation to the isosceles right triangle, it is worth highlighting that the context units, in which this term was discussed, converge (through the semantic criterion of Content Analysis) to a first category entitled ‘construction of the isosceles right triangle’. However, the term perpendicularity, its frequency in the context units, points (through the semantic criterion of Content Analysis) to a related category 'notion of perpendicularity based on the configuration of the instrument' and to another 'positioning of the triangle perpendicularly to board'.
Thus, according to these categories that organize the data, in continuation with the Content Analysis method, hereafter the third stage is presented (treatment of results, inference, and interpretation).
Results and discussion
The analysis is based on the transcription of students' oral communication during laboratory practice as its primary source. In this way, the meaning is considered for possible interpretations and inferences. It is discussed, respectively, about: notion of perpendicularity; construction of the isosceles right triangle; and positioning the triangle perpendicular to the board.
Notion of perpendicularity based on the instrument configuration
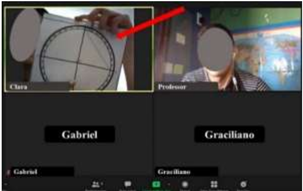
At the beginning of the practice, as already mentioned, the students received as a guide only the student's sheet of paper, which described the guidelines for the practice and Pedro Nunes' instructions for the construction of the instrument jacente no plano. In this document, it was not available a figure that showed the physical configuration of the apparatus, this fact, added to just a first reading of the excerpts from the Cosmographer, made it possible to raise the following question “[...] teacher, we have to draw a triangle on top of the print?” (Clara, 2021, Audio recording Room 3). This same interpretation appears in other rooms, and in this regard, we have (Figure 4).
In response to Clara's questioning and representation, the group was instructed to read the excerpts again in more detail, so that they could reach a conclusion closer to the correct positioning of the triangle. Given the new reading, a member of the group states: “[...] I understand, I understand, since he wants to be perpendicular, it is as if the triangle was standing on the thing, is it standing on the paper, you know? He wants it perpendicular to the circumference! Is it right, teacher? (Gabriel, 2021, Audio recording Room 2). Here the expression “was standing on the thing” appears, which indicates that this idea of standing is one of the meanings he attributes to the concept of perpendicularity.
Taking into account that the teaching material must serve as a mediator to benefit the teacher/student/knowledge relationship in the construction of knowledge (Passos, 2012), then, as a way of encouraging group work using the instrument, Gabriel's question is passed on to the other members of the group, one of them then responds: “[...] I understood, here's the thing, you build the triangle and then bounce it on top of the plane, then raise the triangle, it will serve as a base to build the triangle and lift it up to be perpendicular to the plane, [...] as if it were a raft” (Graciliano, 2021, Audio recording Room 2). Given this interpretation, Flora (2021) states “[...] that was what you were saying Gabriel”. Gabriel concludes his thought by highlighting that:
That! [...] it’s to build the triangle using this circumference, because you will use the two radii as if they were two catheti. Then, using this as if it were a kind of ruler, then you just leave the little triangle standing up, then this triangle that is standing up has to be above the center, because it will be measured in relation to the Sun, right. It is going to be like a sundial (Gabriel, 2021, Audio recording Room 2).
In the speech of these participants, it is possible to observe that in order to understand the configuration of the instrument they attribute meaning to the notion of perpendicularity based on already known concrete objects. The raft, as well as the Sundial, in fact have an upright structure on a base that may indicate perpendicularity. This association made by students from something concrete to the abstract indicates that they still do not have an effective understanding of the concept of perpendicularity, because “[...] for people who have already conceptualized these objects, when they hear the name of the object, it flows in their minds the idea corresponding to the object, without needing the initial support they had from the attributes size, color, movement, shape, and weight” (Lorenzato, 2012, p. 22). What can be seen in the students' speech is that the aforementioned concept is still closely linked to the support of a concrete nature.
In Primary and Secondary Education textbooks, it is possible to observe the appreciation given to concrete support. To start dealing with the topic, in the particular case of perpendicularity, in volume two of the 2016 collection 'Conexões com a matemática' - which means Connections with Mathematics” - from the publisher Moderna, they highlight the gnomon (a stick stuck in the ground to mark shadows) and the plumb line to build walls (Leonardo, 2016). This allusion to concrete supports seen in this collection is in accordance with what Lorenzato (2012) argues, in which these initial supports are seen as elements to awaken a first conceptualization of the objects.
Still on moments of the meeting that allude to the notion of perpendicularity, see Figure 5.
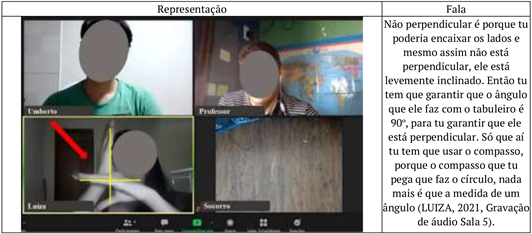
Source: Authors’ archive (Room 5, 2021).
Figure 5 Gestural representation of the notion of perpendicularity.
Focusing on what Luiza says about perpendicularity, it is noted that she brings yet another meaning to compose the concept, since it is known that “[...] geometric representations can be given through drawings, constructed objects, gestures, through language, among other manifestations” (Passos, 2000, p. 39). From what she says, if the triangle is slightly inclined it is not perpendicular to the base, she then uses her hands to represent her argument. The positioning given to the hands actually references the notion of perpendicularity, as it is known that “[...] two straight lines are perpendicular when they intersect forming four congruent angles; each of them is called a right angle” (Lima, Carvalho, Wagner, & Morgado, 2016, p. 177). Her speech also corroborates this fact, since it is said that the angle made by the triangle with the board is 90º.
Given these discussions about perpendicularity based on the configuration of the instrument, students arrive at the representation in Figure 6.
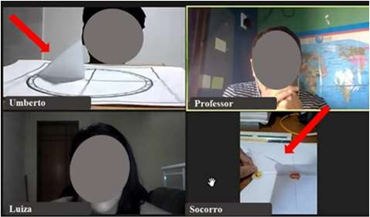
Source: Authors’ archive (Room 5, 2021).
Figure 6 Configuration of the instrument jacente no plano perceived by the students.
As it can be seen, by reading Pedro Nunes' excerpts, the students were able to understand how the triangle should be positioned. However, it is interesting to note that even though they were in the same group (Room 5), they still positioned the triangle differently on the circumference. Note that Umberto places the 90º angle of the isosceles right triangle close to the center of the circle, while Socorro places it at a point on the circumference. When asked about the reason for this impasse, one of the students replies, in a tone of affirmation “Socorro, you put the sides of the triangle wrong” (Luiza, 2021, Audio recording Room 5). It is known according to the excerpts from Pedro Nunes that the way Socorro did it was correct, however, instead of saying that one was right and the other wrong, the students were instructed to do a new reading of the text available on the student’s worksheet.
In the next category, emphasis is given to the way students thought about constructing the triangle.
Construction of the isosceles right triangle
As already mentioned in the previous category, the students' first initiative was to draw the triangle on the graduated circumference. After understanding that the triangle should be perpendicular, they thought about using the circumference “[...] to build the triangle [...], because you will use the two radii as if they were two catheti. Then using this as if it were some kind of ruler” (Gabriel, 2021, Audio recording Room 2). As justification for this, they state “[...] we said that the semidiameter would be the radii and we formed the isosceles right triangle” (Flora, 2021, Audio recording in the main room with all students).
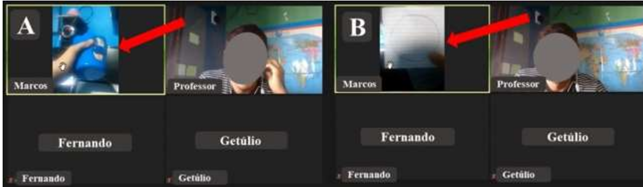
Still in the same direction, another student highlights that “[...] I saw that the most correct method was to draw a straight line (a string) from zero to ninety on the circumference” (Marcos, 2021, Audio recording at main room with all students). However, one of the students' difficulties in using the circumference as a kind of template, as they perceived, was the fact that some students did not have the material previously requested for practice. In order to alleviate this ‘problem’, one of the students uses a sheet and a cylindrical-shaped object to construct the circumference (Figure 7).
In Figure 7 (A) there is the cylindrical object and in Figure 7 (B) the created circumference. Regarding this construction, Marcos (reporter from room 4) highlights that “[...] I built this circle here, with a circular object, I don’t know if it was deformed, but I built it and supposedly drew the diagonals because there is no way to guarantee it” (Marcos, 2021, Audio recording in the main room with all students). The incorporation of the cylindrical object for the construction of the instrument jacente no plano was not foreseen for this practice, however, it is known that “[...] materials need to be integrated into situations that lead to reflection and systematization, so that it starts a formalization process” (Base Nacional Comum Curricular [BNCC], 2018, p. 276).
In these terms, it is understood that the cylindrical object played an important role in the fulfillment of the proposed activity, since in the student's speech it is observed that he is aware of the possible inaccuracy of the material used, and that from it he may not have constructed an exactly circular figure and it is difficult to determine its center to trace the diameters.
Faced with this possible inaccuracy, in order to get closer to something exact, a student asks for the floor and declares that “[...] the construction of the triangle, we make by taking the compass, making a quarter of a circle that is 90º, then the little 90º vertex of the triangle is exactly at what would be the center of the circumference” (Luiza, 2021, Audio recording in the main room with all students). This construction, it seems, involved the transport of angles, as the 90º angle (quadrant) was possibly constructed from the measurements of the semidiameter of the circumference placed on the board (Carvalho, 1958), as this is a very viable construction that we recurrently observed in books on geometric constructions.
Another strategy for constructing the isosceles right triangle, still associated with the circumference, refers to the use of a rigid material designed by the students in Room 1 (Figure 8).

Source: Authors’ archive (Main Room, 2021).
Figure 8 Proposal for constructing the isosceles right triangle.
In this case, it is noted that to construct the triangle, students start from the idea of perpendicularity as a 90º angle. The use of a sheet of paper, a rigid material illustrated with the corners of a notebook, indicates that the students possibly visualize a rectangle on the sheet/cover of the material, which they certainly know has four right internal angles. According to Pais (1996), when taking intuitive, experimental, and theoretical aspects of geometric knowledge as a basis, which are central in an epistemological theory of geometry, it is understood that this notebook serves as a first representation of the concept in the process of apprehending the object.
In the main room, still discussing the construction of this triangle, Marcos asks for the floor to highlight that:
[...] There was a discussion in my group, we had difficulties regarding what an isosceles triangle was, I had understood that they were two equal sides and one smaller, but in fact it is just two equal sides, the other not necessarily smaller or larger, because been isosceles has nothing to do with angles, it has to do with the size of the side, right (Marcos, 2021, Audio recording in the main room with all students).
In this regard, it is noted that the discussion possibly helped them reflect on the concept of isosceles triangle. As the first idea for constructing the triangle was associated with the graduated circle, in this case the triangle formed has one larger side (hypotenuse) and two smaller sides (radii). However, if it were not a right-angled, and only isosceles, then the triangle could have had a pair of congruent larger sides and a smaller one, as Marcos points out.
Given the reflections conducted so far, it is understood that the students had the possibility of reconfiguring and/or expanding the mathematical knowledge mobilized, as the situations promoted the communication of mathematical ideas and the exchange of ideas through group work. (Rêgo & Rêgo, 2012).
Positioning the triangle perpendicular to the board
During the discussions in the main room, with the return of all groups of students, it became clear that Pedro Nunes' instruction to position the triangle perpendicular to the board was the most difficult for them. The proof of this is that only one team managed to present a strategy that was exposed and refined to all participants in the laboratory practice. When presenting the idea, the reporter from Room 5 highlights that:
To ensure that the triangle was perpendicular, we even thought of an argument, but we do not know if it's quite correct. The board has these two diameters here where each one forms 90º [...] then the triangle is on one of these lines, these diameters, on the other diameter we draw a plane, for example if we glued a sheet, we would have to ensure that this angle here is 90º, so it would be perpendicular to it, and we also guarantee this by comparing the angle of the triangle that we know it is 90º with this angle, we will determine that it is equal using the compass (Luiza, 2021, Audio recording in the main room with all students).
At the heart of this strategy, it is noted that the group proposes to work based on the diameters of the circumference. As auxiliary resources, they include the incorporation of a sheet of paper representing a plane and a compass to ensure a 90º angle responsible for the perpendicularity of the isosceles right triangle. In this regard, we have the Figure 9.
In Figure 9, the student represents the triangle with her hand and with a sheet of paper the supposed plane that her team suggested as a strategy. In the words of the reporter “[...] here is the triangle and the other plane that you drew, which is not the board, this plane is on top of the board, you guarantee that it is perpendicular because it is exactly on the other diameter of the board” (Luiza, 2021, Audio recording in the main room with all students). It can be seen that the students are working with perpendicular planes, one represented by the triangle and the other represented by the sheet of paper. In this way, in fact, it is possible to ensure that the triangle would be perpendicular, as the intersection of these planes is a straight line that intersects the board in at least two right angles (one from the triangle and the other from the sheet), which is enough to ensure perpendicularity.
Explaining the idea of Room 5, the student also highlights that “[...] so, we actually have three planes, we have the board, [...] this triangle is contained in another plane too, right, we want to prove that the plane that contains the triangle” (Luiza, 2021, Audio recording in the main room with all students). When asked if the triangle could be considered a plane, Marcos says:
If we considered the piece of paper to be a plane (the graduated circumference) the triangle can also be considered a plane. [...] there are two points of it in a plane and a third is not contained in it, so it means that they form a secant plane, [...] because the straight line is in one and the straight line is outside (Marcos, 2021 , Audio recording in the main room with all students).
In fact, the student's justification is pertinent and acceptable. Pedro Nunes does not provide any information in this regard. He only says that an isosceles right triangle must be constructed in a hard material. It is understood that the cosmographer only refers to the representation of what a triangle would be, as it is evident that whatever material the triangle is constructed from, it will always be, in fact, a right triangular prism and the instrument board a rectangular parallelepiped, this due to the dimensions that indicate spatiality (length, width, and height).
After a request from some colleagues, the reporter of Group 5 returns to talk about her room's strategy. About this, in Figure 10, we seek to detail the student's speech through images.
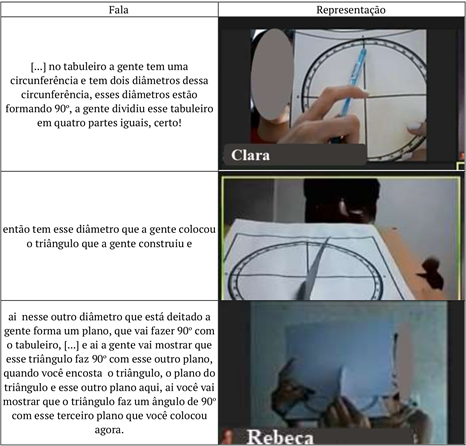
Source: Authors’ archive (Main room, 2021).
Figure 10 Representation of the student's speech about the strategy used.
Given this situation, the idea and validity of the strategy adopted can be clearly observed. It is possible to see the secant planes highlighted previously by Marcos, one of them represented by the triangle and the other by the sheet of paper incorporated to achieve the desired positioning. However, it is worth noting that this construction highlighted by the reporter in Room 5 maintains a mistake, already observed previously, even when they were in internal activity in the group, which is the positioning of the right triangle on the semidiameter. It is known that the 90º vertex of the triangle must be on the circumference, and not at the center. This problem was resolved through a new reading of Pedro Nunes' excerpts and through the reporter's dialogue with the other students from the other groups.
Still on this strategy thought up by the participants in Room 5, concerned about one of the resources used, a student expresses her impression:
- I understand until when she talks about the plane, right. But the idea of the compass confuses me at the end (Rebeca).
- The idea of the compass is just to ensure that the triangle is 90º, because when you have a compass, you form a circumference. And then, let's suppose, if I draw from here to here, I'm drawing the value of an angle, this here is an angle when I draw on the compass. If I take the compass and instead of drawing a whole circle I draw a quarter of a circle, I am drawing a 90º angle. So, I'm going to use the compass to show that I can draw an angle of exactly 90º making a quarter of a circle, did you understand (Luiza).
- But you draw it on another plane? (Rebeca).
- That! (Luiza) (Dialogue among students in the main room, 2021).
In these terms, it is understood that the idea of the compass is to construct a quarter circle in the plane represented by the sheet of paper. This quarter circle, according to the reporter, does not necessarily need to have its sides congruent to the semidiameter of the circumference, as it is mandatory for the triangle that must be placed perpendicular. Given this, it is clear that it would also be valid to just build another triangle and cut it out of the sheet of paper. As for a possible student learning about the concepts covered, the dialogues indicate that this has possibly been achieved, since according to Lorenzato (2012), one of the main triggers for this to happen is the mental activity. In this laboratory activity, learning was present at all times, proof of this are the speeches and actions of the participants in their attempt to comply with Pedro Nunes' instructions. Given this strategy, another idea arises for the positioning of the isosceles right triangle, in relation to it, we have Figure 11.

Source: Authors’ archive (Dialogue among students in the main room, 2021).
Figure 11 Dialogue on the strategy that incorporates the parabolic movement.
In this idea, the student uses the pen as a way to represent the triangle. Thus, it is clear that the student is attributing another meaning to the concept of perpendicularity. Here it appears associated with the highest point of a parabolic movement curve. Furthermore, they also enable them to mobilize/articulate different concepts, not just internal to mathematics itself. Due to time, this strategy was not explored with the students and the activity ended with a brief review of the students' observations presented in order to formalize the triangle positioning strategy.
Considering the students' strategies and actions in order to solve the practical problem driven by Pedro Nunes' instructions, we agree with Pereira and Saito (2019) when they highlight that working with mathematical instruments enables relationships and geometric concepts synthesized in the apparatus gain even more meaning for students.
Thus, thinking about the training of undergraduate Mathematics Education students, it is understood that this practice may have contributed to their understanding that “[...] Geometry involves the study of a broad set of concepts and procedures necessary to solve problems in the physical world and in different areas of knowledge” (BNCC, 2018, p. 271), as some concepts were mobilized in a practical and articulated way.
Considering the theoretical and methodological fundaments, it is understood that the BPU developed possibly favored student learning, as it was permeated by constant exploration and investigation, as predicted by Lorenzato (2012) for the activities that take place within the MTL. The BPU also enabled students to observe mathematical knowledge in practical terms, a potential that meets what was proposed by Miguel and Mendes (2010).
Final considerations
In view of what was observed in the formative action, it is understood that the potential of the instrument for the teaching and learning process lies in placing an understanding of the concepts of isosceles right triangle and perpendicularity in space that are synthesized in it as a necessity for the construction of the instrument. It is this need that actively involves the teacher and students in the teaching and learning process, as it is what motivates students during the formative action and is also responsible for making the MD an instrument of reflection and evaluation.
The fact that students had the possibility of building their own instrument jacente no plano made laboratory practice a lively situation, in which the MD assumed the role of mediator of the student/teacher/knowledge relationship. As some concepts were mobilized in a practical way, they possibly gained more meaning for the students, the same can be said about the possibility of observing how different concepts relate to each other. Having said that, it is noted that the didactic potential of the instrument is not on itself, or its physical form, but rather about the operations that can be conducted with it.
In these terms, it is understood that the instrument jacente no plano can be incorporated into the MTL, as another resource available to the teacher to help develop practices in which they want to think, create, or build mathematical knowledge. For further research, it is worth noting that there is still a lot to be explored regarding the instrument's potential. Here, for example, only the construction of the triangle and its positioning on the board were highlighted. There is still a lack of initiatives to explore the graduation of the circumference and its use in a practical situation of measuring the height of the Sun, and likewise there is a lack of studies that seek to explore this MD in Primary and Secondary Education.
REFERENCES
Almeida, B. J. M. G. P. (2011). A influência da obra de Pedro Nunes na náutica dos séculos XVI e XVII: um estudo de transmissão de conhecimento (Tese de Doutorado). Universidade de Lisboa Faculdade de Ciências Secção Autónoma de História e Filosofia das Ciências, Lisboa. [ Links ]
Bardin, L. (2011). Análise de conteúdo. São Paulo, SP: Edições 70. [ Links ]
Base Nacional Comum Curricular [BNCC]. (2018). Educação é a Base. Brasília, DF: MEC/CONSED/UNDIME. [ Links ]
Canas, A. J. D. C. (2011). A obra náutica de João Baptista Lavanha (c. 1550 - 1624) (Tese de Doutorado). Universidade de Lisboa Faculdade de Letras Departamento de História, Lisboa. [ Links ]
Carvalho, B. A. (1958). Desenho geométrico. Rio de Janeiro, RJ: Ao livro técnico. [ Links ]
Cohen, E. G., & Lotan, R. A. (2017). Planejando o trabalho em grupo (3a ed.). Porto Alegre, RS: Instituto Sidarta. [ Links ]
Creswell, J. W. (2010). Projeto de pesquisa: método qualitativo, quantitativo e misto (3a ed., M. Lopes, Trad.). Porto Alegre, RS: Artmed. [ Links ]
Farias, C. A., & Mendes, I. A. (2014). As culturas são as marcas das sociedades humanas. In I. A. Mendes, & C. A. Farias (Org.). Práticas socioculturais e educação matemática (p. 15-48). São Paulo, SP: Livraria da Física. [ Links ]
Leitão, H. (2008). Anotações ao De arte atque ratione nauigandi. In Pedro Nunes. Obras (p. 515-794, Vol. IV). Lisboa, PT: Academia das Ciências de Lisboa, Fundação Calouste Gulbenkian. [ Links ]
Leonardo, F. M. (2016). Conexões com a matemática (Vol. 2.). São Paulo, SP: Moderna. [ Links ]
Lima, E. L., Carvalho, P. C. P., Wagner, E., & Morgado, A. C. (2016). A matemática do ensino médio (Vol. 2). Rio de Janeiro, RJ: Sociedade Brasileira de Matemática. [ Links ]
Lorenzato, S. (2012). Laboratório de ensino de matemática e materiais didáticos manipuláveis. In S. Lorenzato (Org.), O laboratório de ensino de matemática na formação de professores (p. 57-76, 3a ed.). Campinas, SP: Autores Associados. [ Links ]
Martins, J. P. (2021). Uma linguagem geométrica singular refletida no Códice Atlântico de Leonardo da Vinci: contribuições para a geometria escola (Tese de Doutorado). Universidade Federal do Pará, Instituto de Educação Matemática e Científica, Belém. [ Links ]
Mendes, I. A. (2001). Ensino da matemática por atividades: uma aliança entre o construtivismo e a história da matemática (Tese de Doutorado). Universidade Federal do Rio Grande do Norte. Natal. [ Links ]
Mendes, I. A. (2008). Tendências metodológicas no ensino de matemática. Belém, PA: UFPA. [ Links ]
Mendes, I. A. (2009). Investigação histórica no ensino da matemática. Rio de Janeiro, RJ: Moderna. [ Links ]
Mendes, I. A. (2015). História da matemática no ensino: entre trajetórias profissionais, epistemologias e pesquisas (1a ed.). São Paulo, SP: Livraria da Física . [ Links ]
Miguel, A., & Mendes, I. A. (2010). Mobilizing histories in mathematics teacher education: memories, social practices, and discursive games. ZDM Mathematics Education, 42, 381-392. DOI: https://doi.org/10.1007/s11858-010-0255-8 [ Links ]
Nunes, P. (2008). Obras: De Arte Atque Ratione Navigandi (Vol. IV). Lisboa, PT: Fundação Calouste Gulbenkian. [ Links ]
Oliveira, F. W. S. & Pereira, A. C. C. (2020). Sobre a navegação portuguesa do século XVI à luz do instrumento jacente no plano. Boletim da Sociedade Portuguesa de Matemática, 78, 93-108. [ Links ]
Oliveira, F. W. S. (2019). Sobre os conhecimentos geométricos incorporados na construção e no uso do instrumento jacente no plano de Pedro Nunes (1502-1578) na formação do professor de matemática (Dissertação de Mestrado). Instituto Federação de Educação, Ciência e Tecnologia do Ceará, Fortaleza. [ Links ]
Oliveira, F. W. S. (2021). Sobre a Linguagem Matemática de professores em formação inicial. Boletim Cearense De Educação E História Da Matemática, 8(23), 1108-1121. DOI: https://doi.org/10.30938/bocehm.v8i23.5029 [ Links ]
Pais, L. C. (1996). Intuição, experiência e teoria geométrica. Zetetike, 4(2), 65-74. DOI: https://doi.org/10.20396/zet.v4i6.8646739 [ Links ]
Passos, C. L. B. (2000). Representações, interpretações e prática pedagógica: a geometria na sala de aula (Tese de Doutorado). Faculdade de Educação, Universidade Estadual de Campinas, Campinas. [ Links ]
Passos, C. L. B. (2012). Materiais manipuláveis como recursos didáticos na formação de professores de matemática. In S. Lorenzato (Org.). O laboratório de ensino de matemática na formação de professores (p. 77-92, 3a ed.). São Paulo, SP: Autores Associados. [ Links ]
Pereira, A. C. C., Pinheiro, A. C. M., & Santos, J. N. dos. (2021). A concepção de laboratório de matemática de licenciandos: repensando conceitos, uso e formação.Educação Matemática Em Revista,26(73), 24-43. DOI: https://doi.org/10.37001/emr.v26i73.2915 [ Links ]
Pereira, A. C. C. & Saito, F. (2019). Os conceitos de perpendicularidade e de paralelismo mobilizados em uma atividade com o uso do báculo (1636) de Petrus Ramus. Educação Matemática Pesquisa, 12(1), 405-432. DOI: https://doi.org/10.23925/1983-3156.2019v21i1p405-432 [ Links ]
Rêgo, R. M., & Rêgo, R. G. (2012). Desenvolvimento e uso de materiais didáticos no ensino de matemática. In S. Lorenzato (Org.), O laboratório de ensino de matemática na formação de professores (3a ed., p. 39-56). São Paulo, SP: Autores Associados . [ Links ]
Resolução nº 2, de 20 de dezembro de 2019. (2019, 20 dezembro) Define as Diretrizes Curriculares Nacionais para a Formação Inicial de Professores para a Educação Básica e institui a Base Nacional Comum para a Formação Inicial de Professores da Educação Básica (BNC-Formação). Brasília, DF: CNE/CP. [ Links ]
Turrioni, A. M. S. (2004). O laboratório de educação matemática na formação inicial de professores (Dissertação de Mestrado). Universidade Estadual Paulista, Rio Claro. [ Links ]
11For details on the conception of working from the LAbMatEN of UECE and from some work already performed, see Pereira, Pinheiro, and Santos (2021).
12This formative action is based on the guidelines of the BNC-Formação from the CNE/CP (Resolution nº 2, 2019). The intention is to create an environment that will enable the development of the skills necessary for future professional practice in Primary and Secondary Education.
13A possible translation: “The Works of Peter Nonius of Salacia”. This document consists in a publication in which the author propagates his contributions to support navigation.
14For more information and details on these works of Pedro Nunes, see Almeida (2011), Canas (2011), Leitão (2008), and Oliveira (2019).
15In this work, emphasis is given to this configuration of the instrument jacente no plano, however, it should be known that it can be built differently with some variations. For details on all possibilities, see Oliveira (2019).
16To explore the instrument jacente no plano, the activity was supported by the Resolution Nº 3.599.527 and by the CAAE: 19561119.6.3001.5534), and it had the acceptance of all participants.
17About the name of the participants, considering the ethical aspects of the research, as a way of preserving identity, all names indicated in this research are fictitious.
Received: January 04, 2022; Accepted: June 08, 2022










 texto em
texto em