Servicios Personalizados
Revista
Articulo
Compartir
Revista Brasileira de Educação Especial
versión impresa ISSN 1413-6538versión On-line ISSN 1980-5470
Rev. bras. educ. espec. vol.25 no.2 Marília abr./jun 2019 Epub 31-Mayo-2019
https://doi.org/10.1590/s1413-65382519000200005
Research Report
An Exploratory Study on Strategies and Errors of a Student with Autism Spectrum Disorder when Solving Partitive Division Problems
2Departament of Matemáticas Estadística y Computación, Facultad de Ciencias, Universidad de Cantabria. Avda de los Castros s/n, 39005, Santander (Spain). irene.polo@unican.es. ORCID: http://orcid.org/0000-0001-6425-6337.
3Department of Matemáticas Estadística y Computación, Facultad de Ciencias, Universidad de Cantabria. Avda de los Castros s/n, 39005, Santander (Spain). mariaj.gonzalez@unican.es. ORCID: http://orcid.org/0000-0003-2519-5812.
4Department of Análisis Matemático, Universidad de La Laguna. 38200 San Cristóbal de La Laguna, S/C de Tenerife (Spain). abruno@ull.edu.es. ORCID: http://orcid.org/0000-0002-0154-8073.
In recent years there has been an increasing interest in studying the academic performance of students with Autism Spectrum Disorder (ASD). Among school subjects, mathematics is one of the great obstacles that face students with ASD. It is therefore crucial to go in depth into the understanding that they develop on mathematical concepts, to later provide learning instructions adapted to their needs. This paper explores the strategies and errors that an 11-year old student diagnosed with ASD shows when solving partitive division word problems. A teaching sequence has been designed that includes problems in two different formats: with and without support material. The data was collected during 15 one-hour sessions in which the student solved a total of 49 problems. Results show a clear preference for the one-to-many correspondence strategy in the problems with support material whereas the student mainly resorted to the sharing one-by-one strategy when he did not have the material. A list of errors has been identified related to the meaning of the notions of partition, equity and representativeness, required in partitive division word problems.
KEYWORDS: Special Education; Autism; Problem solving; Strategies; Errors
En los últimos años ha aumentado notablemente el interés por analizar el rendimiento académico de los estudiantes con trastorno del espectro autista (TEA). Entre las materias escolares, las matemáticas son uno de los grandes obstáculos que encuentran estos estudiantes. Por consiguiente, es fundamental mejorar nuestro conocimiento sobre el modo en que los estudiantes con TEA aprenden diferentes conceptos matemáticos para luego proporcionarles métodos de enseñanza adaptados a sus necesidades. Este documento explora las estrategias y los errores que un estudiante de 11 años diagnosticado con TEA muestra al resolver problemas aritméticos verbales de división. Se diseñó una secuencia de enseñanza compuesta por problemas en dos formatos diferentes: con y sin material manipulativo. Se recogieron datos durante 15 sesiones de una hora en las cuales el estudiante resolvió un total de 49 problemas. Los resultados muestran una clara preferencia por la estrategia de reparto por múltiplos para los problemas en los que dispone de material manipulativo, mientras que recurre principalmente a la estrategia de reparto uno a uno cuando no dispone de material. Se identifica un conjunto de errores relacionados con los significados de las nociones de partición, equidad y representatividad, necesarios para resolver con éxito problemas aritméticos verbales de división partitiva.
PALABRAS-CLAVE: Educación Especial; Autismo; Resolución de problemas; Estrategias; Errores
1 Introduction
In the last two decades, many studies have focused on teaching students with learning difficulties to solve mathematical problems (Jitendra, DiPipi, & Perron-Jones, 2002; Montague, 1992). Others have focused on strategic development of arithmetic operations (Siegler, 1988; Zhang, Xin, & Si, 2011). Studies addressing these aspects in students with Autism Spectrum Disorder (ASD), however, are lacking. Several recent studies provide systematic reviews of Mathematics interventions for individuals with ASD (Gevarter et al., 2016; Hart & Cleary, 2015; King, Lemons, & Davidson, 2016). They show that most studies are focusing on the additive structure (Rockwell, Griffin, & Jones, 2011), with few considering the multiplicative structure (Holifield, Goodman, Hazelkorn, & Heflin, 2010; Levingston, Neef, & Cihon, 2009), and almost none dealing with the division operation explicitly (Levingston et al., 2009).
Some of the cognitive traits of students with ASD directly interfere with mathematical problem solving. For example, due to their frequent limitations in understanding the vocabulary or the real situation to which the problem statement refers, these students can have difficulties identifying the arithmetic operation required to solve the problem. Similarly, due to their executive function disorder (planning, organization, impulse control, working memory, etc.), they can have difficulties implementing the actions needed to solve the problem (Hart & Cleary, 2015).
In order to help students with ASD to deal with these problems, many instruction models have focused on teaching metacognitive skills. For example, Whitby (2012) achieved the goal of teaching students with this disorder to solve mathematics problems by memorizing and applying the seven cognitive steps of the “Solve It! Problem-solving Routine” (read, paraphrase, visualize, hypothesize, estimate, compute and check) combined with metacognitive strategies (Montague, 1992). Another study (Gomes, 2007) shows improvement in addition and subtraction skills that were taught to an adolescent with ASD, using procedures derived from Applied Behavior Analysis. Other strategies rely on the use of schematic diagrams to teach students to discriminate and solve types of mathematical word problems. This is the case of numerous studies like those by Rockwell et al. (2011), Kasap and Ergenekon (2017) and Root, Henning, and Boccumini (2018), who successfully followed the so-called Schema-Based Strategy Instruction for teaching arithmetic word problems to students with ASD.
One notable teaching sequence that combines the above ideas is Concrete-Representational-Abstract - CRA (Peterson, Mercer, & O’shea, 1988). In this sequence, physical objects are handled during the Concrete stage to solve word problems. In the Representational stage, images and models are used to represent the objects that appear in the problem. And in the Abstract stage, numbers and mathematical symbols are used. In the case of students with disabilities, this type of progression has been shown beneficial for teaching word problems and other different mathematical notions. For example, Stroizer, Hinton, Flores and LaTonya (2015) used a CRA methodology to teach addition and subtraction involving regrouping and numerical facts involving multiplications to three students with ASD improving their conceptual comprehension of these operations. The work by Yakubova, Hughes and Shinaberry (2016) has shown the effectiveness of a video modeling intervention with CRA instructional sequence when teaching mathematics concepts to students with ASD. Some other intervention studies have successfully used virtual and concrete manipulatives to teach students with ASD arithmetic facts (Bouck, Satsangi, Doughty, & Courtney, 2014) and problem solving (Root, Browder, Saunders, & Lo, 2016).
Given that there is a deficiency in the knowledge of how students with ASD solve division problems, we present an exploratory case study that aims to describe the strategy characteristics and difficulties that a student with ASD shows when solving division problems. In particular, we analyze the strategies that he displays and the observed errors associated to the difficulties he encounters that are related to the conceptual structure of division. We hope that the findings might provide some insight into future instruction designs for students with similar characteristics to those of the participant of the study.
2 Division word problems
Learning an arithmetic operation is a complex process that spans across a long period of time. In the specific case of division the formalization of the operation must be delayed until students develop knowledge of the meanings of this operation (Boero, Ferrari & Ferrero, 1989). To construct this process of learning arithmetic operations, different learning methodologies rely on giving students arithmetic word problems that describe a contextualized situation in a verbal information format. In the case of the division operation, there are two types of problems: quotative and partitive division problems (Fischbein, Deri, Nello, & Marino, 1985). In quotative division problems, the unknown is the number of containers filled: “We have 20 pieces of candy and want to put four pieces of candy in each bag. How many bags do we need?”. In partitive division problems, the unknown is the number of objects that each container has: “We have 20 pieces of candy and want to distribute them into five bags. How many pieces of candy go in each bag?”. In this study, we will focus on partitive division problems.
There is little research about the way in which persons with ASD learn division. One exception is the work by Levingston et al. (2009), which evaluates a learning method for multiplication and division problems based on recurrent behaviors in two subjects, one of whom has ASD (Gevarter et al., 2006; King et al., 2016). That work, however, does not examine the meaning that the students assign to these problems in terms of their structure (partitive division or quotative division), nor does it describe the strategies they used, which underscores the need to carry out studies in this direction.
2.1 Strategies for solving division problems
Research about strategies with students with Mathematics difficulties has usually focused on the additive structure, and there is a shortage of research that analyzes how the multiplicative reasoning develops (Zhang et al., 2011). Furthermore, there are no studies addressing these aspects that consider students with ASD (Gevarter et al., 2016; Hart & Cleary, 2015; King et al., 2016). We are particularly interested in those studies that focus on the representations used and on the steps taken by the students. Taking these facets into account, the following three levels for solving a partitive division problem are described (Downton, 2008; Mulligan, 1992):
Direct modeling with counting. Concrete objects or drawings are used to represent the action described in the problem. The students form equal sets from the quantity given in the problem and arrive at the solution by counting. Different strategies may be used at this level: (1) Sharing one by one: the student distributes objects one by one in the containers and counts the number of objects in each container at the end. (2) One-to-many correspondence: the student assigns a number of objects to each container and at the end verifies that all of the objects have been used. If they have not, he repeats the process using a different initial estimate for the number of objects. (3) Trial and error grouping: the objects are distributed at random among the containers and then the number in each group is adjusted to achieve an equal distribution.
No direct modeling. The same steps as in the previous level are taken, but objects are not used; instead, the student develops the verbal aspects and solving processes of the problem.
Known or derived facts. Reiterated mental addition/subtraction or multiplication operations are carried out to obtain the result of the division.
Typically-developing students exhibit some of the above strategies more often than others, and, in many cases, they follow a progressive pattern (Downton, 2008). For example, the majority of studies agree in their findings that the sharing one by one strategy is the least frequent, and that in those cases where this strategy is exhibited, the students quickly progress to more efficient strategies based on estimates and on one-to-many correspondence (Mulligan, 1992). It has also been shown that low achieving students usually use fewer strategies than high achieving students, and that they show difficulties in transitioning from intuitive strategies to advanced strategies (Siegler, 1988). Our study aims to contribute by examining what peculiarities the strategies described above have in a student with ASD.
2.2 Conceptual structure in partitive division problems
In order to solve an arithmetic word problem, students have to use reading comprehension skills and must be able to transform the word and numbers into the correct operations. Persons with ASD tend to process auditory and/or linguistic information more slowly than typically-developing individuals, albeit within a high range of variability. They may also find it complex to process verbal and visual information simultaneously (American Psychiatric Association [APA], 2000)
The underlying conceptual structure of partitive division includes the idea of partitioning a quantity into parts (the total is divided into two pairwise disjoint parts), along with the ideas of equity (all of the split parts are equal) and the representativeness of each part (any one part is representative of the rest) (Correa, Nunes, & Bryant, 1998). Thus, in order to successfully solve a partitive division problem, the students: (1) have to separate the total number of objects (dividend) into containers (divisor) with no reminder; (2) equally; and (3) interpreting each container as being representative of the rest, with the number of objects in said container (quotient) being the solution to the question posed in the problem. When students have difficulties with understanding the conceptual structure of division, they take the wrong actions, which might be revealed by the following errors:
-
Types of error associated with the conceptual structure of partitive division
-
Errors related to the meaning of partition:
-
Error 1.1. Not separating the whole into parts. The students reiterate the total number of objects in each of the containers. Figure 1 (left) shows an example of this error in a 6:3 distribution.
Error 1.2. Distributing more objects than the total. In situations in which the students have available to them more objects than those indicated in the statement (either because they have a large collection of objects or because they are able to draw them), they distribute them equally but place more objects than they should into the boxes (see Figure 1 (center)).
Error 1.3. Distributing fewer objects than the total. The students distribute the objects equally without using all of the objects available (see Figure 1 (right)).
-
-
Errors related to the meaning of equity:
-
Error 2.1. Not distributing the objects equally. The students distribute the total quantity among the containers available, but do not ensure that the resulting groups are identical. Figure 2 (left) shows this error in a 6:3 distribution.
-
-
Errors related to the meaning of each container’s representativeness:
Error 3.1: Leaving empty groups. The students distribute the objects available equally, but ignore one or more containers. This reveals that they do not interpret each container as being representative of the others, with any container being identical to the others. Figure 2 (right) shows this error in a 6:3 distribution.
Error 3.2: Solving it correctly and giving the initial amount as the answer. For example, when distributing 6:3, they place two objects in each container but answer “I have to put six tokens in each box”. We interpret this to mean that they do not realize that the contents of any one of the containers is the solution to the problem.
Error 3.3: Solving it correctly and giving the number of groups as the answer. As with error 3.2, they correctly place two objects in each container but state that “I have to put three tokens in each box”, not realizing that the contents of each container is the solution to the problem.
-
The above errors can be combined when solving a problem. For example, the objects may be distributed unequally, leaving empty groups (errors 2.1 and 3.1). This situation would indicate that the students have difficulties with the idea of both equity and the representativeness of each container. We will use this error categorization just presented to analyze the data from the study we conducted, the objectives, methodology and results of which are presented next.
3 Objectives of the study
In this exploratory study we consider how a student with ASD initially learns division by solving partitive division problems. The problems of the study are presented to the participant in two different formats: with and without support material. In this paper we analyze the development of the strategies distinguishing between these formats, which are intended to develop an understanding of the meanings of this operation. The goal of this study is to describe the key procedures that the student carries out, more specifically:
The characteristics of the strategies and external representations used, in reference to the format in which the problems are presented (with and without support material).
The observed errors associated to difficulties with the conceptual structure of partitive division (the notions of partition, equity and representativeness).
4 Method
4.1 Participant
We followed a descriptive case study methodology involving a student with ASD who will be referred to as Tom. The subject of the investigation is a male of 11 years and 8 months old at the beginning of the study. He was diagnosed with ASD at the age of 6 by a child psychiatrist, based on clinical evaluation and following diagnostic criteria of the Diagnostic and Statistical Manual of Mental Disorders (DSM-IV, APA, 2000). In the clinical evaluation no other comorbidity was found other than the diagnostic of ASD. According to the Global Assessment of Functioning Scale (GAF), the subject presents a level of affection between 41 and 50, characterized by severe symptoms with severe impairment of social, work or school activity.
The student presents a broad repertoire of stereotypical behaviors and exhibits repetitive conduct and an obsession for certain subjects. He has a full scale IQ of 54, as measured by the Wechsler intelligence scale for children WISC V. At the beginning of the study, he scored 56/72 (35/41 in informal thinking and 18/31 in formal thinking) in the test of early math achievement TEMA 3 (Ginsburg & Baroody, 2003), which situates his mathematical knowledge in the second year of Primary school. Tom employs a functional language with a high level of both oral and written comprehension. As a student, he is disciplined and shows a special interest in manual tasks and drawing. Tom follows an adapted mathematics curriculum and takes supplementary language and mathematics classes. The numerical content he had learned prior to our research was: addition and subtraction with regrouping (he has not memorized number facts), solving addition and subtraction problems in writing; multiplication problems, though he had not memorized the times tables.
4.2 Design
In order to evaluate the knowledge of the student about the division operation, an initial test was applied which consisted in partitive and quotative division problems. The student was also able to solve all quotative division problems with drawings, but he was unable to find an answer in any of the partitive division problems, which he solved using multiplicative incorrect strategies through drawings. For this reason, a teaching sequence with partitive division problems was designed. The instruction was provided during supplementary classes in a weekly work session typically lasting one hour. Tom was given two to five problems in each session, depending on the receptiveness he demonstrated during the session. Over the course of three months and 15 sessions, he worked on a total of 49 problems. A Special Education teacher with previous experience with students with this disorder led all the sessions. The data collected in this study correspond to the records of all spontaneous student resolutions when solving problems.
4.3 Instruction
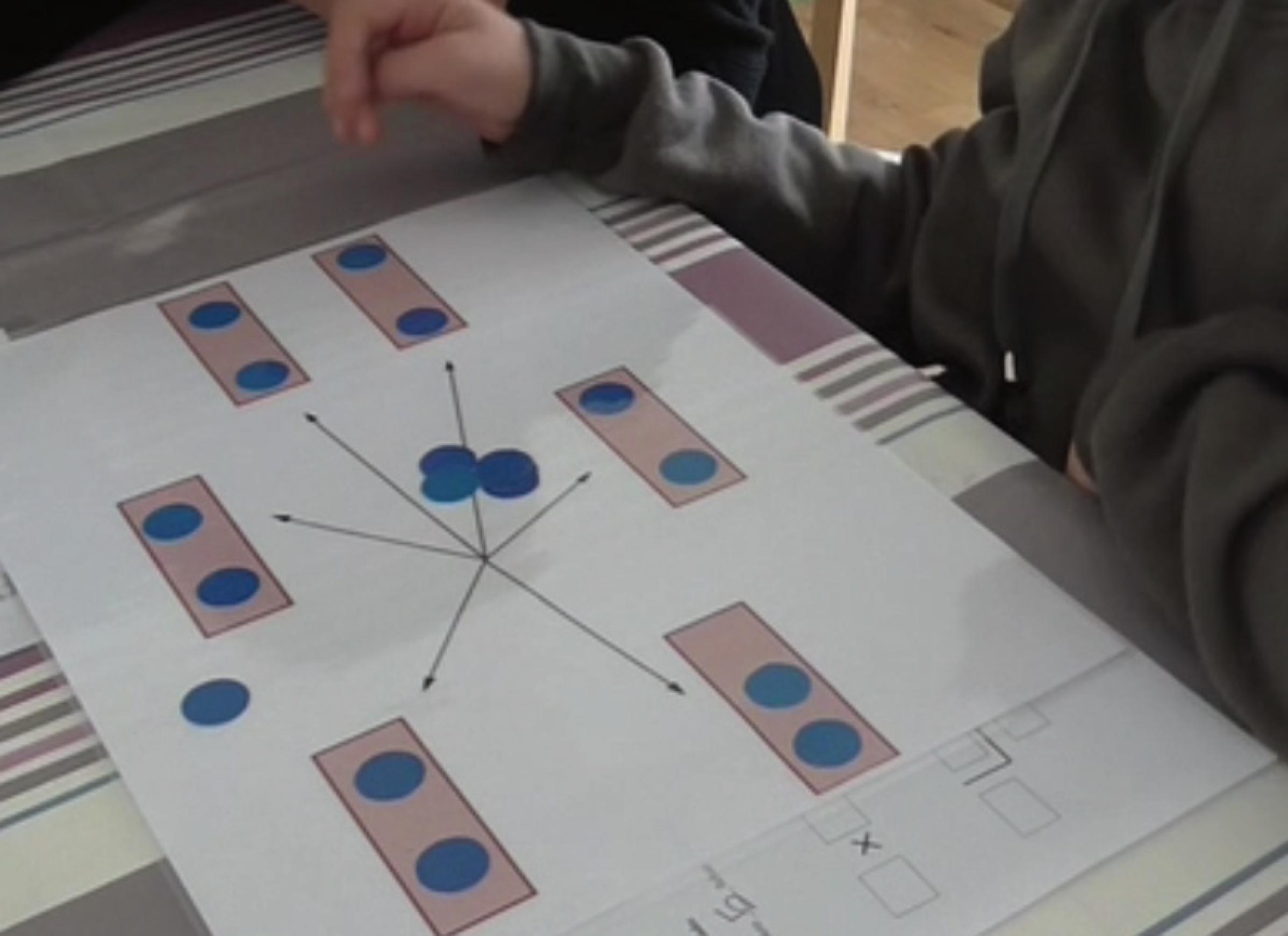
Different studies conducted with students with ASD have shown the benefits of using tangible and visual aids to teach arithmetic operations (Hart & Cleary, 2015; Rockwell et al., 2011). The use of augmentative devices, such as pictograms, also enhances and facilitates communications with individuals with this disorder (Mirenda, 2003). Both conclusions led us to craft a tangible device that resembles a pictogram, and which we call “pictomaterial”. This material is a posterboard with empty rectangular boxes drawn on it and tokens that have to be distributed among the boxes. Also drawn on the cardboard are arrows representing the distribution task (see Figure 3). This material is similar to the schemes used in previous studies conducted on students with ASD (Rockwell et al., 2011), which utilized the Schema-Based Instruction methodology, with the added possibility of being able to manipulate the objects involved in the distribution. Different pieces of plasterboard were made, varying the number of boxes drawn based on the divisor. In the problems where this material was provided, the student was given the sheet of posterboard associated with the divisor in the problem and as many tokens as required for the dividend.
A teaching sequence using partitive division problems was designed. This sequence included problems in two different formats: with and without support material, as follows:
With support material: The problem is presented to the student in written format accompanied by the concrete material mentioned in the problem (such as lollipops and bags) or by the pictomaterial. The pictomaterial was used primarily in the initial sessions and was accepted naturally by the student. The concrete material and the pictomaterial were then interspersed so that the pictographic nature of the latter could allow the student to associate the idea of distribution with a visual scheme.
Without support material: The problem is presented to the student in written format with no support of concrete material or pictomaterial (initially the problem texts were accompanied by a drawing to represent the divisor, which was later removed).
All problems used in the study were partitive division problems, where the total and the number of recipients were known quantities. Problems included names and contexts familiar to the student to make them interesting to him. The size of the total amount (dividend) ranged from 8 to 28. An example of such a problem is: Peter wants to share 20 pencils equally into 4 boxes. How many pencils does he put in each box? Each problem was provided written on a sheet of paper containing the problem statement and a box for Tom to write the final result, thus allowing us to observe if he was able to interpret if the number he obtained was the solution to the problem.
In the first session, the method for solving the problems was explained to Tom, with the pictomaterial being used to explain the distribution task. This way, Tom started to associate this action with the meaning of the word “distribute”. In successive sessions, and following teaching methodologies of previous studies of informal strategies (Mulligan, 1992; Zhang et al., 2011), the tutor always let Tom solve each problem by himself so as to observe his strategies without intervening in them. When Tom encountered difficulties that he was unable to solve by himself, the tutor made a demonstration with pictomaterial showing how to share the total amount equally into the boxes. An example of such a situation is shown below.
As shown in the extract of the instruction, the student first shows an error of not separating the total into parts (error 1.1) and repeating the total amount in each container. With the help of the pictomaterial, he selects the total (8 tokens) and makes a distribution, first leaving empty containers, and finally solving it correctly. Table 2 shows the type and sequence of the 49 problems presented over the course of the 15 sessions.
Table 1 Example of teaching situation using pictomaterial.
| Problem: Janet wants to share 8 lollipops equally into 4 glasses. How many lollipops does she put in each glass? |
|---|
| Tutor: Now read the next problem. You can write and draw anything you want to solve it. Tom: [He draws 4 glasses, and then 8 lollipops in each of the glasses. He writes “she puts 8 lollipops”]. Tutor: Are you sure? How many lollipops have you drawn in total? Tom: [after counting them] 32. Tutor: And how many lollipops does Janet have to share? Tom: 8. Tutor: Why don’t you use this to solve the problem? [she hands him the pictomaterial with divisor four]. Tom: [taking 8 tokens from the pictomaterial, he begins to share them into the four boxes. First he places 3 tokens in each of the first two boxes and keeps the remaining two in his hand. He looks confused]. Tutor: Look carefully, do all boxes have tokens? Tom: No [he starts again placing 2 tokens in each box of the pictomaterial]. Tutor: Very good! Why don’t you do it now on the worksheet? Tom: [He draws the four glasses, two lollipops in each of the four glasses, and writes the correct answer]. |
| Session | S1 | S2 | S3 | S4 | S5 | S6 | ||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Problem | P1 | P2 | P1 | P2 | P3 | P1 | P2 | P1 | P2 | P3 | P1 | P2 | P3 | P1 | P2 | P3 |
| Stage | MP | MP | MP | MC | MC | MP | MC | MC | MP | NM | MP | NM | NM | MC | MP | NM |
| Session | S7 | S8 | S9 | S10 | |||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Problem | P1 | P2 | P3 | P1 | P2 | P3 | P1 | P2 | P3 | P4 | P5 | P1 | P2 | P3 | P4 |
| Stage | MC | NM | NM | MP | NM | NM | MP | NM | NM | NM | MC | NM | NM | NM | MC |
Table 2
Distribution of problems by session based on materials provided. MP: problem with pictomaterial, MC: problem with concrete material, NM: problem without material.
4.4 Data collection
The data were collected during the sessions described. The answers to the problems that Tom solved by hand were gathered and all of the sessions were video recorded. This material was analyzed independently by three researchers, who for each problem identified: (1) The type of strategy used, based on the types specified above: sharing one by one, one-to-many correspondence and trial and error grouping; (2) the errors associated with the meaning of partitive division, based on the error types identified above, and related with the notions of partition, equity and the representativeness of each container; and (3) the correctness of the final answer. The analysis conducted by the three researchers were then compared, and they identified those cases where their interpretations did not match and analyzed them again together. The student tried to solve some of the problems multiple times. The information on each of these attempts was collected. The tutor also noted aspects of the student’s attitude and behavior prior to and during the session. This information was used to omit some of the sessions in which the student seemed to have a lack of concentration. The omitted sessions were repeated when the student was more receptive to them. The inter-rater reliability (obtained by the formula agreements/(agreements + disagreements) x 100%) across all sessions was 97%.
5 Results
Below we present the characteristics of the strategies used by the student and the errors identified as he solved partitive division word problems, based on the format in which the problem is presented to the student distinguishing between: (1) With material support: problems without drawing with support from the concrete material or pictomaterial; and (2) Without material support: problems with no material aids.
5.1 Strategy characteristics and types of errors when solving problems with material support
The student used the materials offered for every problem presented in this format, both the concrete material and the pictomaterial. The three distribution strategies identified by Mulligan (1992) were exhibited: (1) one-to-many correspondence, (2) sharing one by one, and (3) trial and error grouping, described above. Table 3 shows the progression in the types of strategies used over the course of the 15 sessions with material aids, distinguishing between correct and incorrect answers.
Table 3 Strategies used in problems with support material (with concrete material or pictomaterial). TEG: Trial and error grouping; OMC: One to many correspondence; SOO: Sharing one-by-one.
| CORRECT | SOO | 1 | 1 | 2 | 1 | 1 | ||||||||||||
| OMC | 1 | 2 | 1 | 1 | 2 | 1 | 1 | |||||||||||
| TEG | 1 | |||||||||||||||||
| INCORRECT | SOO | 1 | ||||||||||||||||
| OMC | 1 | 1 | 2 | 1 | ||||||||||||||
| TEG | 1 | |||||||||||||||||
| Sessions | S1 | S2 | S3 | S4 | S5 | S6 | S7 | S8 | S9 | S10 | S11 | S12 | S13 | S14 | S15 | |||
(1) As it is shown in the table, the one-to-many correspondence (OMC) strategy was preferred by the student for the problems with concrete materials. In the first three sessions, he had some problems, though he eventually overcame them. He did not make any subsequent adjustments in any of the cases, meaning he directly selected the quotient as the initial group. This was interpreted to mean he was doing some kind of preliminary mental summation calculation that allowed him to obtain the quotient in a simple manner when the numbers involved were small. When this strategy yielded incorrect results, it was either because he picked an initial quantity that was smaller than the result, leaving undistributed elements, or because he picked an initial quantity that was larger than the result, leaving some containers empty. We associate the first case with a difficulty with the meaning of partition, thought to stem from the fact that he has not yet developed the idea that this type of distribution requires not leaving any items undistributed (error 1.3). In these cases, the student began the grouping and stopped before all the objects were distributed. For example, when distributing 18 tokens in six boxes with pictomaterial, Tom began grouping them by twos and stopped after distributing 12 tokens, regarding the problem as solved (see Figure 3).
We associate the second case, leaving containers empty, with a difficulty understanding each container’s representativeness (error 3.1), since he divides the total and places an equal number of objects in some containers, which he fills, while ignoring one or more of the remaining containers. This indicates that he is cognizant of the need to distribute the total number of objects equally, but without realizing that any container is representative of any other container.
(2) The next most common strategy used in the problems presented with material format was sharing one-by-one (SOO). This strategy yielded the correct solution in every case, with one notable exception that took place in one of the early sessions, where the student correctly used it to distribute six items in three containers, but wrote as the answer “There are three in each box”. We interpret that the error made in this case resulted from giving the number of groups as the answer instead of the contents of any container (error 3.3).
(3) Only rarely did the student use the trial and error grouping (TEG) strategy with material aids (3 out of 24). In another of these cases, Tom made a subsequent adjustment, correctly distributing six objects in three boxes, but he wrote the final answer as “There are six objects in each box”. In this case, the error resulted from giving the initial number as the answer instead of the contents of any container (error 3.2).
The progress made in the problems with material aids during the sessions reveals an initial stage in which Tom used all three strategies with some difficulties, preferring the use of the one to many correspondence strategy. From session 6 onwards, he correctly solved all the problems with materials, with two distinguishable patterns: the continued use of one-to-many correspondence through session 11, and from there until session 15, the use of sharing one-by-one. As we will see next, he systematically adopted this latter strategy, probably as a result of the success he was having in problems with no material aids.
5.2 Strategy characteristics and types of errors when solving problems without support material
The problems presented in a format with no material aids were introduced in the fourth session, and Tom also employed the three distribution strategies: (1) one to many correspondence, (2) sharing one-by-one, and (3) trial and error grouping. Table 4 shows the progression in the strategies used by Tom for the problems with no material aids, and the number of attempts for each strategy.
Table 4 Strategies used in problems with no support material. TEG: Trial and error grouping; OMC: One to many correspondence; SOO: Sharing one-by-one.
| CORRECT | SOO | 1 | 2 | 2 | 3 | 2 | ||||||||||
| OMC | 1 | 1 | 3 | 2 | 1 | |||||||||||
| TEG | 1 | 1 | 1 | |||||||||||||
| INCORRECT | SOO | 1 | 2 | |||||||||||||
| OMC | 1 | 1 | 1 | 2 | 3 | 3 | ||||||||||
| TEG | 1 | 2 | 1 | |||||||||||||
| Sessions | S1 | S2 | S3 | S4 | S5 | S6 | S7 | S8 | S9 | S10 | S11 | S12 | S13 | S14 | S15 | |
(1) Initially, the student used the one to many correspondence strategy (OMC), but by way of a new multiplicative procedure in which he drew the total amount to distribute in each container instead of separating the total into groups (see Figure 4). We interpret this error as a manifestation of the difficulty understanding the notion of partition: the student does not associate the idea of distribution with the complete allocation into groups (error 1.1). Logically, this difficulty could only be exhibited in formats with no material aids due to the possibility of being able to draw as many objects as desired.
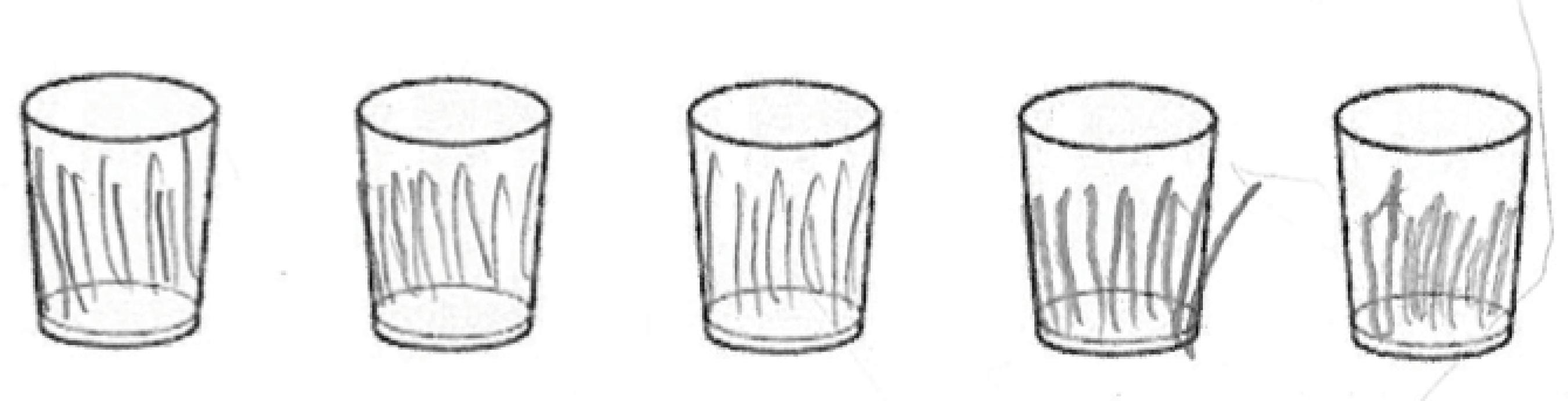
Figure 4 One to many correspondence strategy. Error 1.1 - Not separating the whole amount into parts (10:5 division).
To overcome this difficulty, the tutor invited him to use the pictomaterial and to select the total amount of tokens to be shared (see Table 1 for an example of such a teaching situation) and, later, to replicate the resolution in the worksheet. Using this one to many correspondence strategy, supplemented with a preliminary drawing of the amount to distribute, the student made several mistakes. Figure 5 shows three attempts at solving the same 12:4 division problem. In the first attempt, he distributes more objects than there are in the quotient (four instead of three), filling every container and increasing the total number of items to distribute (error 1.2). In the second attempt, he also selects a larger number of objects that is higher than the quotient, but adheres to the total amount, as a result leaving a container empty (error 3.1). The last attempt shows the correct solution.

Figure 5 One to many correspondence strategy. Error 1.2 - Distributing more objects than the total (left). Error 3.1 - Leaving groups empty (center). Right answer (right) (12:4 division).
(2) The trial and error grouping strategy (TEG) in problems with no material aids was rarely used, and was combined with the one to many correspondence strategy. For example, Figure 6 shows two attempts at solving an 8:4 division problem. In the first attempt, the student drew 11 pencils before erasing two of them and distributing the remaining nine into three of the glasses using a trial and error strategy. Two errors are present: distributing more objects than the total (error 1.2) and leaving groups empty (error 3.1). In the second attempt, he correctly solved the problem by using a one to many correspondence strategy. Note the variation in the level of detail in the representation of the objects in the first attempt (concrete representation) versus the second attempt (schematic representation).
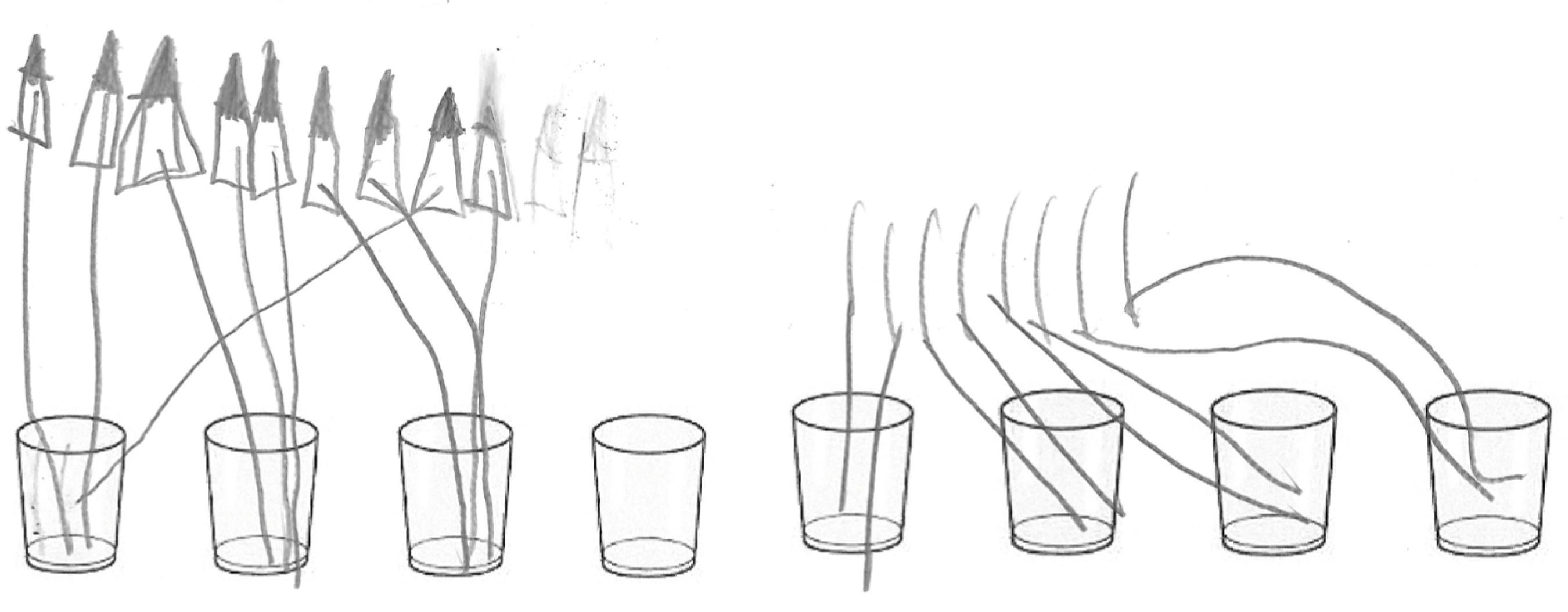
Figure 6 Trial and error strategy. Error 1.2 and 3.1.(distributing more objects and leaving empty groups) (left). One to many correspondence strategy (correct answer) (right) (8:4 division).
Another example in which the trial and error strategy was used allowed us to identify a problem with the idea of equity (error 2.1). In Figure 7, we can see that in a 30:5 distribution, Tom places the tokens arbitrarily in the containers until there are no more left, without verifying that every container has the same amount of tokens.
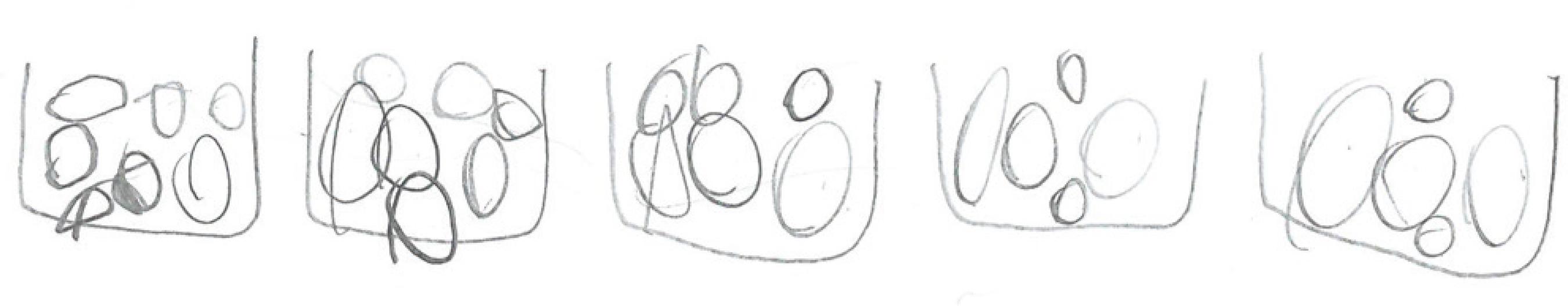
Figure 7 Trial and error strategy. Error 2.1 - Not distributing the objects equally (30:5 division).
(3) The student finally adopted a sharing one-by-one strategy (SOO), which helped him overcome the errors he had made. He successfully implemented this strategy in all problems with and without support material of the last two sessions.
As Table 4 shows, many of the initial attempts were unsuccessful, and are associated primarily with the one to many correspondencestrategy. From session 11, he mainly resorted to the sharing one-by-one strategy, which proved successful and which he applied to problems with material aids as well, as noted in the previous section (see Table 3).
5.3 Types of errors identified in terms of the solution strategy
From the standpoint of the conceptual structure underlying the partitive division operation, Table 5 shows the frequency of the errors encountered and the strategies associated with the format of the problem (with and without support material).
Table 5 Frequency of errors by format (with and without support material) and strategy type. TEG: Trial and error grouping; OMC: One to many correspondence; SOO: Sharing one-by-one.
| Conceptual structure | Error type | With material | Without material | ||||
|---|---|---|---|---|---|---|---|
| SOO | OMC | TEG | SOO | OMC | TEG | ||
| Meaning of partition | Error 1.1. Does not separate the total | - | - | - | - | 5 | - |
| Error 1.2. Distributes more than the total | - | - | - | 1 | 2 | - | |
| Error 1.3. Distributes less than the total | - | 1 | - | - | - | - | |
| Meaning of equity | Error 2.1. Objects not distributed equally | - | - | - | 1 | - | 3 |
| Meaning of each container representativeness | Error 3.1. Leaves groups empty | - | 2 | - | 1 | 3 | 1 |
| Error 3.2. Gives initial amount | - | 3 | 1 | - | 1 | - | |
| Error 3.3. Gives number of groups | 1 | - | - | - | - | - | |
The student exhibited different errors in all of the strategies utilized (see Table 5), though the majority occurred when using the one to many correspondence strategy. In the problems with material aids, almost all of the errors were associated with ignoring the representativeness of any container as the solution to the problem, while in the problems with no material aids, the difficulties stemmed from ignoring the requirement to make the distribution equal and from two aspects involving the idea of partition (not separating the whole amount into parts and distributing more objects than the total).
6 Discussion and conclusions
After the entire process, we see a positive progression in the resolution of the problems, but with a significant difference in the strategies used by the student depending on the format in which the problem was presented (with and without the support of material). The use of concrete materials and/or pictomaterials provided essential support to the student, who naturally employed a one to many correspondence strategy from the start, and only rarely the trial and error strategy. Once the student undertook the sharing one by one strategy, he seemed to realize the success of this strategy since he adopted it in problems with materials, despite its inefficiency in problems with large numbers. The order in which the strategies appeared in the case of the student involved in this study differs from what is known for typically-developing students, who tend to use sharing one-by-one strategies when they first learn division before subsequently developing more efficient strategies based on one to many correspondence (Mulligan, 1992). This preference for a more rudimentary strategy could be related to the inclination exhibited by some students with ASD to resort to mechanical methods that offer them more comfort (Bae, 2013).
We identified different errors, distinguishing the ones based on the absence of meaning in the notions of partition, equity and each container’s representativeness. The reported errors show difficulty in understanding the underlying conceptual structure of this operation and the way in which quantities relate to each other. They might also be linked to language difficulties, characteristic of people with ASD (APA, 2000; Bae, 2013). For instance, they might be associated with difficulty in interpreting the meaning of certain keywords of sharing situations (like each, share or equally…). However, the present study does not provide strong evidence regarding these connections, and the use of other forms of data like verbal or non-verbal behaviour might be required in future research to make such connections.
Although it was not one of the focuses of this study, it is worth noting the role that the representations made by the student have played in the resolutions. We noted a preference for detailed, realistic drawings in most of the solutions, frequent in some individuals with ASD (Booth, Charlton, Hughes, & Happé 2003). This occasionally distracted Tom, diverting his attention from the data and questions in the problem and impeding the solution process. In light of these results, it seems necessary to leverage the strength that some individuals with autism spectrum disorder exhibit in terms of their enjoyment of drawing, but we must find ways to use it effectively so as to motivate them to solve the problem, without the details in the drawing standing in the way of the solution process (for example by using simple objects in the problem statement, whose concrete representation does not differ significantly from a schematic representation: sticks, lollipops…).
The results of this exploratory study provide information on the division learning process in a student with ASD, and suggest methodological approaches for helping students with characteristics similar to those of the study participant to successfully solve partitive division problems. This study has provided evidence that a student with ASD can solve a variety of division problems by using informal strategies before learning formal ones (based on number facts and the division algorithm). It is important for teachers of students with ASD to consider these pre-formalization strategies, as they provide a basis for the subsequent learning of arithmetic operations (Mulligan, 1992). Carpenter and Moser (1984) found that many typically-developing children, after learning formal arithmetic procedures, would sometimes do the problems methodically, with no understanding. Though we are unaware of findings in students with ASD, we can take steps to prevent this from happening.
A careful observation of the errors by students with ASD when using the initial strategies can help identify which aspects of the meaning of division to emphasize at any given moment during instruction (partition, equity and representativeness). This is important since, as has been shown, mistakes are not the result of randomness or student carelessness; rather, they reflect conceptual problems with the operation. The use of manipulatives (pictomaterial or the like) is one means for helping students with ASD to understand the meanings of words normally used in division problems. The physical actions and the orientation of the motions with the objects help to connect with the vocabulary involved: “each”, “equal” or “distribute”. At the same time, the use of these materials provides the instructor with the necessary feedback who can see how the student integrates these meanings, thus the materials becoming a non-verbal method of communication.
As in many studies with students with learning difficulties, we followed a case study methodology that does not allow for generalization, but that can serve as a basis for subsequent research with individuals affected by this disorder.
Acknowledgment
This work was supported by the Ministerio de Economía y Competitividad - Madrid (Spain), Resolución de problemas y competencia matemática en la educación primaria y secundaria y en la formación de profesores (EDU2017-84276-R).
REFERENCES
American Psychiatric Association (2000). Diagnostic and statistical manual of mental disorders (4th ed., text rev.). Washington, DC: Author. [ Links ]
Bae, Y. S. (2013). Word Problem Solving of Students with Autistic Spectrum Disorders and Students with Typical Development (Ph.D. Dissertation). Columbia University. ProQuest. [ Links ]
Boero, P., Ferrari, P. L. y Ferrero, E. (1989). Division Problems: Meanings and Procedures in the Transition to a Written Algorithm. For the Learning of Mathematics, 9, 17-25. [ Links ]
Booth, R., Charlton, R., Hughes, C. y Happé, F. (2003). Disentangling weak coherence and executive dysfunction: Planning drawing in Autism and ADHD. Philosophical Transactions of the Royal Society, 358, 387-392. [ Links ]
Bouck, E. C., Satsangi, R., Doughty, T. T. y Courtney, W. T. (2014). Virtual and concrete manipulatives: A comparison of approaches for solving mathematics problems for students with autism spectrum disorder. Journal of Autism and Developmental Disabilities, 44, 180-193. [ Links ]
Carpenter, T. P. y Moser, J. M. (1984). The acquisition of addition and subtraction concepts in grades one through three. Journal for Research in Mathematics Education, 15, 179-203. [ Links ]
Correa, J., Nunes, T. y Bryant, P. (1998). Young children’s understanding of division: the relationship between division terms in a noncomputational task. Journal of Educational Psychology, 90, 321-329. [ Links ]
Downton, A. (2008). Links between children’s understanding of multiplication and solution strategies for division. En M. Goos y K. Makar (Eds.), Proceedings of the 31st annual conference of the Mathematics Education Research Group of Australasia (pp. 171-178). Sydney, Australia: Mathematics Education Research Group of Australasia. [ Links ]
Fischbein, E., Deri, M., Nello, M. y Marino, M. (1985). The role of implicit models in solving verbal problems in multiplication and division. Journal for Research in Mathematics Education, 16, 3-17. [ Links ]
Gevarter, C., Bryant, D. P., Bryant, B., Watkins, L., Zamora, C. y Sammarco, N. (2016). Mathematics interventions for individuals with autism spectrum disorder: A systematic review. Review Journal of Autism and Developmental Disorders, 3, 224-238. [ Links ]
Ginsburg, H. P. y Baroody, A. J. (2003). Test of Early Math Achievement (3rd ed.). Austin, TX: Pro-Ed. [ Links ]
Gomes, C. G. S. Autismo e ensino de habilidades acadêmicas: adição e subtração. Revista Brasileira de Educação Especial, 13, 345-364. [ Links ]
Hart, J. E. y Cleary, S. (2015). Review of evidence-based mathematics interventions for students with autism spectrum disorders. Education and Training in Autism and Developmental Disabilities, 50, 172-185. [ Links ]
Holifield, C., Goodman, J., Hazelkorn, M. y Heflin, L. J. (2010). Using self-monitoring to increase attending to task and academic accuracy in children with autism. Focus on Autism and Other Developmental Disabilities, 25, 230-238. [ Links ]
Jitendra, A., DiPipi, C. M. y Perron-Jones, N. (2002). An exploratory study of schema-based word problem solving instruction for middle school students with learning disabilities: An emphasis on conceptual and procedural understanding. Journal of Special Education, 36, 23-38. [ Links ]
Kasap, C. y Ergenekon, Y. (2017). Effects of a schema approach for the achievement of the verbal mathematics problem-solving skills in individuals with autism spectrum disorders. Educational Sciences: Theory & Practice, 17, 1787-1809. [ Links ]
King, Seth A.; Lemons, Christopher J.; Davidson y Kimberly A. (2016). Math Interventions for Students with Autism Spectrum Disorder: A Best-Evidence Synthesis. Exceptional Children, 82, 443-462. [ Links ]
Levingston H. B., Neef, N. A y Cihon, T. M. (2009). The effects of teaching precurrent behaviors on children’s solution of multiplication and division word problems. Journal of Applied Behavior Analysis, 42, 361-367. [ Links ]
Mirenda, P. (2003). Toward functional augmentative and alternative communication for students with autism: manual signs, graphic symbols, and voice output communication aids. Language, Speech, and Hearing Services in Schools, 34, 203-216. [ Links ]
Montague, M. (1992). The effects of cognitive and metacognitive strategy instruction on mathematical problem solving of middle school students with learning disabilities. Journal of Learning Disabilities, 25, 230-248. [ Links ]
Mulligan, J. (1992). Children’s solutions to partition problems. En B. Southwell, R. Perry y K. Owens (Eds.), Proceedings of the 15th Annual Conference of the Mathematics Education Research Group of Australasia (pp. 410-420). Sydney: MERGA. [ Links ]
Peterson, S. K., Mercer, C. D. y O’Shea, L. (1988) Teaching learning disabled students place value using the concrete to abstract sequence. Learning Disabilities Research, 4, 52-56 [ Links ]
Rockwell, S. B., Griffin, C. C. y Jones, H. A. (2011). Schema-Based Strategy Instruction in Mathematics and the Word Problem-Solving Performance of a Student with Autism. Focus on Autism & Other Developmental Disabilities, 26, 87-95. [ Links ]
Root, J. R., Browder, D. M., Saunders, A.F. y Lo, Y. (2016). Schema-Based Instruction with concrete and virtual manipulatives to teach problem solving to students with autism, Remedial and Special Education, 48, 42-52. [ Links ]
Root, J. R., Henning, B. y Boccumini, E. (2018). Teaching students with autism and intellectual disability to solve algebraic word problems. Education and Training in Autism and Developmental Disabilities, 53, 325-338 [ Links ]
Siegler, R. (1988). Individual differences in strategy choices: Good students, not-so-good students, and perfectionists. Child Development, 59, 833-851. [ Links ]
Stroizer, S., Hinton, V., Flores, M. y LaTonya, T. (2015). An investigation of the effects of CRA instruction and students with autism spectrum disorder. Education and Training in Autism and Developmental Disabilities, 50, 223-236. [ Links ]
Whitby, P. J. S. (2012). The effects of “Solve It!” on the mathematical word problem solving ability of adolescents with autism spectrum disorders. Focus on Autism and Other Developmental Disabilities, 28, 78-88. [ Links ]
Yakubova, G., Hughes, E. M. y Shinaberry, M. (2016) Learning with technology: video modeling with concrete-representational-abstract sequencing for students with autism spectrum disorder. Journal of autism and developmental disorders, 46, 2349-2362. [ Links ]
Zhang, D., Xin, Y.P. y Si, L. (2011). Transition from intuitive to advanced strategies in multiplicative reasoning for students with math difficulties, Journal of Special Education, 47, 50-64. [ Links ]
Received: November 28, 2018; Revised: February 28, 2019; Accepted: March 02, 2019










 texto en
texto en